Δύο ημέρες πριν το σεισμό της 26ης Ιανουαρίου στην Κεφαλλονιά, καταγράφησαν ασυνήθη ηλεκτρομαγνητικά σήματα MHz ταυτόχρονα από σταθμούς που είναι εγκατεστημένοι στην Κεφαλλονιά και τη Ζάκυνθο.
Επτά Επιστήμονες από 4 εκπαιδευτικά Ιδρύματα της χώρας, εκπόνησαν και δημοσίευσαν επιστημονική εργασία, σύμφωνα με την οποία διαπιστώνεται με δεδομένα και επιστημονική μεθοδολογία, η παρατήρηση ηλεκτρομαγνητικών μεταβολών γύρω από την Κεφαλλονιά, μόλις 2 ημέρες πριν το σεισμό της 26ης Ιανουαρίου 2014 που έπληξε το νησί μας.
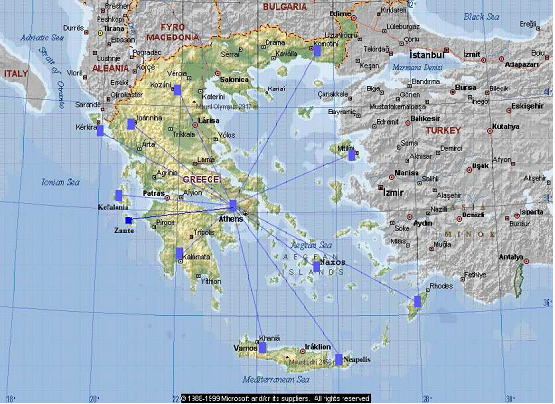
Σε όλη τη χώρα είναι εγκατεστημένοι 11 τηλεμετρικοί σταθμοί παρακολούθησης και καταγραφής των Ηλεκτρομαγνητικών εκπομπών φασματικής περιοχής MHz – kHz. Την 24η Ιανουαρίου 2014, ταυτόχρονα οι σταθμοί της Κεφαλλονιάς και της Ζακύνθου, κατέγραψαν αξιοσημείωτη μεταβολή ηλεκτρομαγνητικών σημάτων.
Αναλύοντας τα δεδομένα που κατέγραψε το τηλεμετρικό δίκτυο παρατήρησης ηλεκτρομαγνητικών εκπομπών, οι Έλληνες επιστήμονες παρατηρούν ότι οι δύο σταθμοί μέτρησης που είναι εγκατεστημένοι στην Κεφαλλονιά και τη Ζάκυνθο αντίστοιχα, κατέγραψαν μεταβολές στις ηλεκτρομαγνητικές εκπομπές. Οι μεταβολές αυτές που ξεφεύγουν των συνήθων καταγραφών, δεν παρατηρήθηκαν σε άλλους σταθμούς της χώρας. Ωστόσο παρατηρήθηκαν ταυτόχρονα από τους σταθμούς της Κεφαλλονιάς και της Ζακύνθου στις 24 Ιανουαρίου 2014, δηλαδή δύο ημέρες πριν το χτύπημα του εγκέλαδου.
Αν και η εργασία των επιστημόνων αποδεικνύει αιτιώδη σχέση μεταξύ των ηλεκτρομαγνητικών σημάτων και του επακόλουθου σεισμού, ωστόσο ενισχύει την επικρατούσα επιστημονική θέση ότι οι ηλεκτρομαγνητικές μεταβολές αποτελούν ένα από τα πρόδρομα φαινόμενα του σεισμού. Από την άλλη πρέπει να τονίσουμε ότι σημαντικές μεταβολές της συχνότητας των ηλεκτρομαγνητικών σημάτων δεν σημαίνουν ότι επέρχεται πάντα σεισμός.
Για την ευρύτερη περιοχή της Κεφαλλονιάς, η ασυνήθης παρατήρηση συνίσταται στην καταγραφή ηλεκτρομαγνητικών εκπομπών συχνότητας MHz, και είναι εμφανής στις παρακάτω απεικονίσεις.
Οι ηλεκτρομαγνητικές καταγραφές στο σταθμό της Κεφαλλονιάς, δύο ημέρες πριν το σεισμό της 26ης Ιανουαρίου
*Η περιοχή μεταξύ των κόκκινων γραμμών στο πάνω αριστερό γράφημα, δείχνει τις πρόδρομες ηλεκτρομαγνητικές μεταβολές.
Οι ηλεκτρομαγνητικές καταγραφές στο σταθμό της Ζακύνθου, δύο ημέρες πριν το σεισμό της 26ης Ιανουαρίου
Η περιοχή μεταξύ των κόκκινων γραμμών δείχνει τις πρόδρομες ηλεκτρομαγνητικές μεταβολές.
Τα 11 σημεία εγκατάστασης του τηλεμετρικού δικτύου καταγραφής ηλεκτρομαγνητικών μεταβολών στην Ελλάδα. Ο μετρητής της Κεφαλλονιάς και ο μετρητής της Ζακύνθου κατέγραψαν ταυτόχρονα παρόμοιες ηλεκτρομαγνητικές μεταβολές, δύο ημέρες πριν από το μεγάλο σεισμό.
Για λόγους αξιοπιστίας του κειμένου μας παραθέτουμε αυτούσια την επιστημονική εργασία όπως δημοσιεύθηκε στην αγγλική γλώσσα.
On the recent M=6.1 earthquake occurred at Kefalonia island (South-West Greece) on 26 January 2014: Manifestations of an Earth system in critical state.
Y. Contoyiannis1, S. M. Potirakis2, J. Kopanas3, G. Antonopoulos3, G. Koulouras4, K. Eftaxias1, C. Nomicos4.
1. Department of Physics, Section of Solid State Physics, University of Athens, Panepistimiopolis, GR-15784, Zografos, Athens, Greece, (Y. C.: yconto@yahoo.gr, K. E.: ceftax@phys.uoa.gr)
1. Department of Electronics Engineering, Technological Education Institute (TEI) of Piraeus, 250 Thivon & P. Ralli, GR-12244, Aigaleo, Athens, Greece, spoti@teipir.gr.
- Department of Environmental Technology and Ecology, Technological Education Institute (TEI) of the Ionian Islands, Panagoulas road, GR-29100, Zante, Greece, (e- J. K.: jkopan@otenet.gr; e- G. A.: sv8rx@teiion.gr)
- Department of Electronics Engineering, Technological Education Institute (TEI) of Athens, Ag. Spyridonos, GR-12210, Aigaleo, Athens, Greece, cnomicos@teiath.gr.
Abstract
In this paper we show, in terms of fracture-induced electromagnetic emissions (EME) recorded two days prior to the earthquake of Kefalonia (Cephalonia), Greece [(38.22o N, 20.53oE), 26 January 2014, M=6.1] that the Earth system around the focal area came to critical condition two days before the earthquake occurence. Specifically, the MHz EME recorded by the remote telemetric stations on the island of Kefalonia and the neighboring island of Zante came simultaneously to critical conditions. The analysis was performed by means of the method of critical fluctuations (MCF) revealing critical features.
Keywords: Fracture-induced electromagnetic emissions, Seismicity, Criticality,
Greece.
1 Introduction
The possible connection of the electromagnetic (EM) activity that is observed prior to significant earthquakes (EQs) with the corresponding EQ preparation processes, often referred to as seismo-electromagnetics, has been intensively investigated during the last years. Several possible EQ precursors have been suggested in the literature [Uyeda et al., 2009a; Cicerone et al, 2009; Hayakawa, 2013a, 2013b]. The possible relation of the field observed fracture- induced electromagnetic emissions (EME) in the frequency bands of MHz and kHz has been examined in a series of publications [e.g., Eftaxias et al, 2001, 2004, 2008; Kapiris et al., 2004; Karamanos et al., 2006; Papadimitriou et al., 2008; Contoyiannis et al., 2005, 2013; Eftaxias and Potirakis, 2013a; Potirakis et al., 2011, 2012a, 2012b, 2012c, 2013; Minadakis et al., 2012a, 2012b], while a three stage model for the preparation of an EQ by means of its observable EM activity has been recently put forward [Eftaxias and Potirakis, 2013b, and references therein].
In this letter, we report the recording of two MHz EM signals, with a sampling rate of 1 sample/s, prior to a recent significant EQ occurred in south-west Greece. On 26 January 2014 (13:55:43 UT) an M = 6.1 occurred on the Island of Kefalonia (Cephalonia), while 2 days before two remote telemetric stations of our remote observation stations network, the station of Kefalonia (located on the same island) and the station of Zante (located on a neighboring island of the same island complex) recorded simultaneously the aforementioned signals. Note that, it has been repeatedly made clear that the pre-EQ EME signals have been recorded only prior to strong shallow EQs that happened in land (or near coast-line); this also, in combination to the recently proposed fractal geo-antenna model [Eftaxias et al., 2004], explains why they manage to be transmitted to the air. This model gives a good reason for the increased possibility of detection of such EM radiation, since it has as a consequence an increased radiated power compared to the power that would be radiated if a dipole antenna model was considered.
The analysis of the specific EM time-series, using the method of critical fluctuations (MCF) [Contoyiannis and Diakonos, 2000; Contoyiannis et al., 2002, 2013], reveals critical features, implying that the possibly related underlying geophysical process is at critical state. The presence of the “critical point” during which two active parts of the system are highly correlated even at arbitrarily long distances, in other words when “everything depends on everything else”, is consistent with the view that the EQ preparation process during the period that the MHz EME are emitted is a spatially extensive process. It is noted that, according to the aforementioned three stage model [Eftaxias and Potirakis, 2013b, and references therein], the pre-seismic MHz EM emission is considered to be originate during the fracture of the part of the Earth’s crust that is characterized by highly heterogeneity. During this phase the fracture is non-directional and spans over a large area that surrounds the family of large high- strength entities distributed along the fault sustaining the system. Note that for an EQ of magnitude ~6 the corresponding fracture process extends to a radius of ~120km [Bowman et al, 1998].
2 Data analysis methods
The analysis of the recorded data was performed using the MCF [Contoyiannis and Diakonos, 2000; Contoyiannis et al., 2002, 2013] and the natural time method [Varotsos et al., 2011; Potirakis et al, 2013, and references therein]. Although they come from difference grounds, both of them manage to reveal the existence of critical behavior by simple statistical time-series processing and the examination of the compliance to specific criteria. Detailed descriptions of all the involved calculations can be found elsewhere for the MCF [Contoyiannis et al., 2013] and the natural time method [Potirakis et al., 2013] and therefore are omitted here for the sake of brevity and focus on the findings. However, general descriptions of the employed methods follow.
MCF was proposed for the analysis of critical fluctuations in the observables of systems that undergo a continuous phase transition [Contoyiannis and Diakonos, 2000; Contoyiannis et al., 2002]. It is based on the finding that the fluctuations of the order parameter, that characterizes successive configurations of critical systems at equilibrium, obey a dynamical law of intermittency of an 1D nonlinear map form. The MCF is applied to stationary time windows of statistically adequate length, for which the distribution of the of waiting times l (laminar lengths) of fluctuations in a properly defined laminar region is fitted by a function f (l)μ Γp2e~p}l. The criteria for criticality are p2 > 1 and p3 » 0 [Contoyiannis
and Diakonos, 2000; Contoyiannis et al., 2002]. In that case the system is characterized by intermittent dynamics, since the distribution follows power-law decay [Schuster, 1998]. On the other hand, in the case of a system governed by noncritical dynamics the corresponding distribution follows an exponential decay, rather than a power-law one [Contoyiannis et al., 2004b]. The MCF has been applied to a variety of dynamical systems, including thermal (e.g., 3D Ising) [Contoyiannis et al, 2002], geophysical [Contoyiannis et al., 2004a; Contoyiannis and Eftaxias 2008; Contoyiannis et al, 2010] and biological systems (electro-cardiac signals) [Contoyiannis et al.,2004b; Contoyiannis et al., 2013].
3 Analysis results
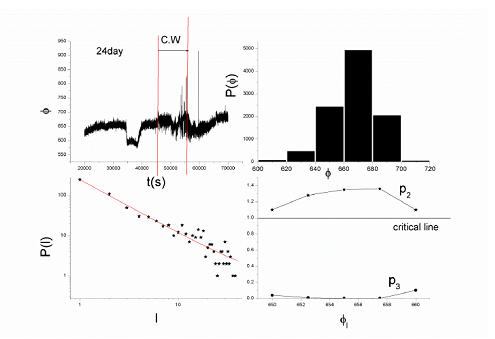
Part of the MHz recordings of the Kefalonia station is shown in Fig. 1a. This was recorded in Julian day 24, that is ~2 days before the occurrence of Kefalonia EQ. This stationary time-series excerpt, having a total length of 2.8h (10000 samples) starting at 24 Jan. 2014 (12:46:40 UT), was analyzed by the MCF method and was identified to be a “critical window” (CW). CWs are time intervals of the MHz EME signals presenting features analogous to the critical point of a second order phase transition [Contoyiannis et al., 2005].
The main steps of the MCF analysis [Contoyiannis et al., 2013] on the specific time- series are shown in Fig. 1b- Fig. 1d. First, a distribution of the amplitude values of the analyzed signal was obtained from which, using the method of turning points [Pingel et al., 1999], a fixed-point, that is the start of laminar regions, fo of about 700mV was determined. Fig. 1c portrays the obtained laminar distribution for the end point f = 655mV, that is the distribution of waiting times, referred to as laminar lengths l, between the fixed-point f0 and the end point f, as well as the fitted function f (l)μ ΓΑepl with the corresponding exponents p2 = 1.35 , p3 = 0.000 with R = 0.999 . Finally, Fig. 1d shows the obtained plot of the p2, p3 exponents vs. f. From Fig. 1d it is apparent that the criticality conditions, p2 > 1 and p3 » 0, are satisfied for a wide range of end points f, revealing the power-law decay feature of the time-series that proves that the system is characterized by intermittent dynamics; in other words, the MHz time-series excerpt of Fig. 1a is indeed a CW.
Fig. 1. (a) The 10000 samples long critical window of the MHz EME that was recorded at the Kefalonia station. (b) Amplitude distribution of the signal of Fig. 1a. (c) Laminar distribution for the end point f = 655mV, as a representative example of the involved fitting. The solid line corresponds to the fitted function (cf. to text in Sec. 2) with the values of the corresponding exponents p2, p3 also noted. (d) The obtained exponents p2, p3 vs. different values of the end of laminar region f. The horizontal dashed line indicates the critical limit ( p2 =1).
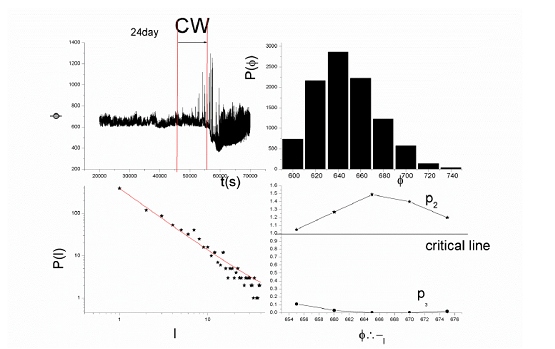
Part of the MHz recordings of the Zante station is shown in Fig. 2a. This was also recorded in Julian day 24, that is ~2 days before the occurrence of Kefalonia EQ. This stationary time-series excerpt, having a total length of 2.8h (10000 samples) starting at 24 Jan. 2014 (12:46:40 UT), was also analyzed by the MCF method and was identified to be a “critical window” (CW).
The main steps of the MCF analysis [Contoyiannis et al, 2013] on the specific time- series are shown in Fig. 2b- Fig. 2d. First, a distribution of the amplitude values of the analyzed signal was obtained from which, using the method of turning points [Pingel et al., 1999], a fixed-point, that is the start of laminar regions, f0 of about 600mV was determined. Fig. 2c portrays the obtained laminar distribution for the end point f = 665mV, that is the distribution of waiting times, referred to as laminar lengths l, between the fixed-point and the end point f, as well as the fitted function f (l)μ ΓΑe p with the corresponding exponents p2 = 1.49, p3 = 0.000 with R = 0.999 . Finally, Fig. 1d shows the obtained plot of the p2, p3 exponents vs. f. From Fig. 1d it is apparent that the criticality conditions, p2 > 1 and p3 » 0, are satisfied for a wide range of end points f, revealing the power-law decay feature of the time-series that proves that the system is characterized by intermittent dynamics; in other words, the MHz time-series excerpt of Fig. 1a is indeed a CW.
Fig. 2. (a) The 10000 samples long critical window of the MHz EME that was recorded at the Zante station. (b) Amplitude distribution of the signal of Fig. 2a. (c) Laminar distribution for the end point f = 665mV, as a representative example of the involved fitting. The solid line corresponds to the fitted function (cf. to text in Sec. 2) with the values of the corresponding exponents p2, p3 also noted. (d) The obtained exponents p2, p3 vs. different values of the end of laminar region f. The horizontal dashed line indicates the critical limit ( p2 =1 ). l
In summary, we conclude that both stations recorded MHz signals that simultaneously presented critical state features two days before the main event.
1 Discussion – Conclusions
Based on the method of critical fluctuations, we have shown that the fracture-induced MHz EME recorded by two stations of our remote observation stations network prior to the recent significant EQ of Kefalonia present criticality characteristics, implying that they emerge from a system in critical state.
There are two key points that render these observations unique in the up to now research on the preseismic EME:
(i) The Kefalonia station is known for being insensitive to EQ preparation processes happening outside of the wider area of Kefalonia island, as well as to EQ preparation processes leading to low magnitude EQs within the area of Kefalonia island. Note that the only signal that has been previously recorded refers to the M=6 EQ that occurred on the specific island in 2007 [Contoyiannis et al., 2010].
(ii) MHz EME presenting critical characteristics were simultaneously recorded in two different stations very close to the focal area, while no other station of our network (cf. Fig. 3) has recorded such signals prior to the specific EQ. This feature, combined with the abovementioned sensitivity of the Kefalonia station only to significant EQs occurring on the specific island, may be considered as an indication of the location of the impending EQ.
We finally note that the multidisciplinary analysis of the kHz EME recorded our stations is currently in progress.
Fig. 3. The 11 remote sensing stations completing the telemetric network for the recording of electromagnetic variations in the MHz and kHz bands in Greece.
Acknowledgements
Research co-funded by the EU (European Social Fund) and national funds, action “Archimedes III—Funding of research groups in T.E.I.”, under the Operational Programme “Education and Lifelong Learning 2007-2013”.
REFERENCES
Bowman, D., G. Ouillon, C. Sammis, A. Sornette, and D. Sornette (1998), An observational test of the critical earthquake concept, J. Geophys. Res., 103, 24359-24372, doi: 10.1029/98JB00792.
Cicerone, R. D., J. E. Ebel, and J. Britton (2009), A systematic compilation of earthquake precursors, Tectonophysics, 476, 371-396, doi: 10.1016/j.tecto.2009.06.008.
Contoyiannis, Y., and F. Diakonos (2000), Criticality and intermittency in the order parameter space, Phys. Lett. A, 268, 286 -292, doi: 10.1016/S0375-9601(00)00180-8.
Contoyiannis, Y., F. Diakonos, and A. Malakis (2002), Intermittent dynamics of critical fluctuations, Phys. Rev. Lett., 89, 035701, doi: 10.1103/PhysRevLett.89.035701.
Contoyiannis, Y. F, F. K. Diakonos, P. G. Kapiris, A. S. Peratzakis, K. A. Eftaxias (2004a), Intermittent dynamics of critical pre-seismic electromagnetic fluctuations, Phys. Chem. Earth, 29, 397-408, doi: 10.1016/j.pce.2003.11.012.
Contoyiannis, Y. F., F. K. Diakonos, C. Papaefthimiou and G. Theophilidis (2004b), Criticality in the relaxation phase of a spontaneously contracting atria isolated from a Frog’s Heart, Phys. Rev. Lett, 93, 098101, doi: 10.1103/PhysRevLett.93.098101.
Contoyiannis, Y.F., P. G. Kapiris, and K. A. Eftaxias (2005), A Monitoring of a pre- seismic phase from its electromagnetic precursors, Phys. Rev. E, 71, 066123, 066123/1-14, doi: 10.1103/PhysRevE. 71.066123.
Contoyiannis, Y. F. and K. Eftaxias (2008), Tsallis and Levy statistics in the preparation of an earthquake, Nonlin. Processes Geophys., 15, 379-388, doi:10.5194/npg-15-379-2008.
Contoyiannis, Y.F., C. Nomicos, J. Kopanas, G. Antonopoulos, L. Contoyianni, K. Eftaxias (2010), Critical features in electromagnetic anomalies detected prior to the L’Aquila earthquake, Physica A, 389, 499-508, doi: 10.1016/j.physa.2009.09.046.
Contoyiannis, Y. F., S. M. Potirakis, and K. Eftaxias (2013), The Earth as a living planet: human-type diseases in the earthquake preparation process, Nat. Hazards Earth Syst. Sci., 13, 125-139, doi: 10.5194/nhess-13-125-2013.
Eftaxias, K., P. Kapiris, J. Polygiannakis, N. Bogris, J. Kopanas, G. Antonopoulos, A. Peratzakis and V. Hadjicontis (2001), Signatures of pending earthquake from electromagnetic anomalies. Geophys. Res. Let., 28, 3321-3324, doi: 10.1029/2001GL013124.
Eftaxias, K., P. Frangos, P. Kapiris, J. Polygiannakis, J., Kopanas, A. Peratzakis, P. Skountzos, and D. Jaggard (2004), Review and a model of pre-seismic electromagnetic emissions in terms of fractal electrodynamics, Fractals, 12, 243-273, doi: 10.1142/S0218348X04002501.
Eftaxias, K., Y. Contoyiannis, G. Balasis, K. Karamanos, J. Kopanas, G. Antonopoulos, G. Koulouras, and C. Nomicos (2008), Evidence of fractional-Brownian-motion-type asperity model for earthquake generation in candidate pre-seismic electromagnetic emissions, Nat. Hazards Earth Syst. Sci., 8, 657-669, doi:10.5194/nhess-8-657-2008.
Eftaxias, K., and S. M. Potirakis (2013a), On the puzzling feature of the silence of precursory electromagnetic emissions, Nat.. Hazards Earth Syst. Sci., 13, 2381-2397, doi: 10.5194/nhess-13-2381-2013.
Eftaxias, K., and S. M. Potirakis (2013b), Current challenges for pre-earthquake electromagnetic emissions: shedding light from micro-scale plastic flow, granular packings, phase transitions and self-affinity notion of fracture process, Nonlin. Processes Geophys.,, 20, 771-792, doi:10.5194/npg-20-771-2013.
Gutenberg, B. (1956), The energy of earthquakes, Quart. J. Geol. Soc. London, 112, 1, doi: 10.1144/GSL.JGS.1956.112.01-04.02.
Hayakawa, M. (ed.) (2013a), The Frontier of Earthquake Prediction Studies, Nihon- Senmontosho-Shuppan, Tokyo.
Hayakawa, M. (ed.) (2013b), Earthquake Prediction Studies: Seismo Electromagnetics, Terrapub, Tokyo.
Kanamori, H. (1977), The energy release in great earthquakes, J. Geophys. Res., 82(20), 2981, doi: 10.1029/JB082i020p02981.
Kapiris, P., K. Eftaxias, and T. Chelidze (2004), Electromagnetic Signature of Prefracture Criticality in Heterogeneous Media, Phys. Rev. Lett., 92(6), 065702/1-4, doi: 10.1103/PhysRevLett.92.065702.
Karamanos, K., D. Dakopoulos, K. Aloupis, A. Peratzakis, L. Athanasopoulou, S. Nikolopoulos, P. Kapiris, and K. Eftaxias (2006), Study of pre-seismic electromagnetic signals in terms of complexity. Phys. Rev. E, 74, 016104/1-21, doi: 10.1103/PhysRevE.74.016104.
Minadakis, G., S. M. Potirakis, C. Nomicos, and K. Eftaxias (2012a), Linking electromagnetic precursors with earthquake dynamics: an approach based on nonextensive fragment and self-affine asperity models, Physica A, 391, 2232-2244, doi: 10.1016/j.physa.2011.11.049.
Minadakis, G., S. M. Potirakis, J. Stonham, C. Nomicos, and K. Eftaxias (2012b), The role of propagating stress waves in geophysical scale: Evidence in terms of nonextensivity, Physica A, 391(22), 5648-5657, doi:10.1016/j.physa.2012.04.030.
Papadimitriou, K., M. Kalimeri, and K. Eftaxias (2008), Nonextensivity and universality in the earthquake preparation process, Phys. Rev. E, 77, 036101/1-14, doi: 10.1103/PhysRevE.77.036101.
Pingel, D., P. Schmelcher and F. K. Diakonos (1999), Theory and examples of the inverse Frobenius-Perron problem for complete chaotic maps, Chaos, 9, 357-366, doi: 10.1063/1.166413.
Potirakis, S. M., G. Minadakis, C. Nomicos, and K. Eftaxias (2011), A multidisciplinary analysis for traces of the last state of earthquake generation in preseismic electromagnetic emissions, Nat. Hazards and Earth Syst. Sci., 11, 2859-2879, doi:10.5194/nhess-11-2859- 2011.
Potirakis, S. M., G. Minadakis, and K. Eftaxias (2012a), Analysis of electromagnetic pre-seismic emissions using Fisher information and Tsallis entropy, Physica A, 391, 300-306, doi:10.1016/j.physa.2011.08.003.
Potirakis, S. M., G. Minadakis, and K. Eftaxias (2012b), Sudden drop of fractal dimension of electromagnetic emissions recorded prior to significant earthquake, Nat. Hazards, 64, 641-650, doi:10.1007/s11069-012-0262-x.
Potirakis, S. M., G. Minadakis, and K. Eftaxias (2012c), Relation between seismicity and pre-earthquake electromagnetic emissions in terms of energy, information and entropy content, Nat. Hazards Earth Syst. Sci, 12, 1179-1183, doi:10.5194/nhess-12-1179-2012.
Schuster, H. (1998), Deterministic Chaos, VCH, Weinheim.
Uyeda, S., T. Nagao, and M. Kamogawa (2009a), Short-term EQ prediction: Current status of seismo-electromagnetics, Tectonophysics, 470, 205-213.